
This means that Γ is constrained to be within this circular space, such that |Γ|≤ 1. (Note that the curve did not change shape, only the axes of the graph did.) It’s useful to note that the magnitude of the reflection coefficient can never exceed 1.0, as this would imply that power is somehow “created” by the load. On this plot, the same green marker is now at a magnitude (denoted as |Γ|) of about 0.2 (i.e., its distance from the center or origin), and a phase angle of about 75°. Reading off the chart, we see the following values: frequencyīecause complex numbers can also be represented in polar form, we can also plot Γ on a polar plot, as in Figure 2. Figure 1, Reflection Coefficient, rectangular form. See Figure 1: the green marker is at 14.175 MHz. Our DUT for this example is a 50-ohm coaxial transmission line terminated by a 40m OCF dipole.įirst, let’s plot the real and imaginary components of Γ for this DUT on a rectilinear graph.
SMITH CHART VSWR DOWNLOAD
We can measure Γ by connecting a VNA to a device under test (DUT), “sweep” a band of interest, and download the data.

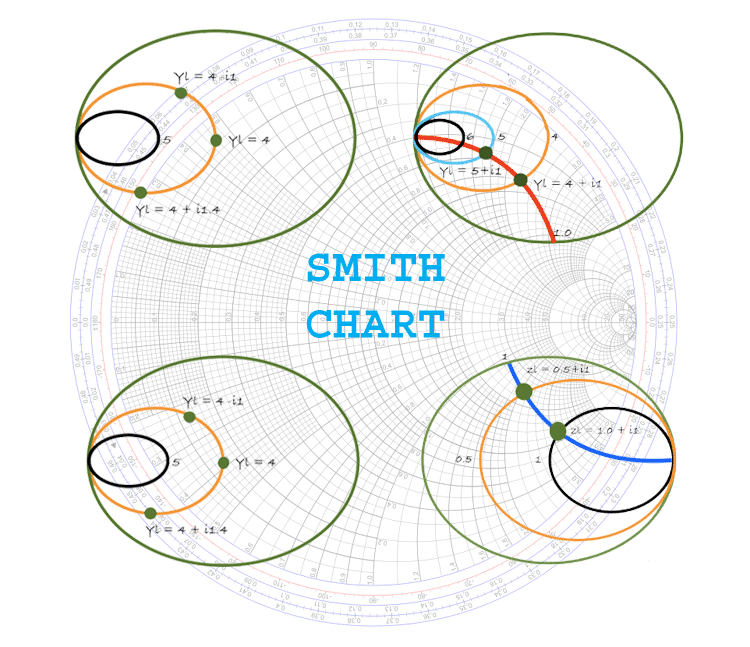
(For a perfect termination, Γ=0, an open termination yields G=1, and a short results in Γ=-1.) Being a complex quantity, Γ can convey both the magnitude and phase of the reflected wave, at a particular frequency. Notated using the Greek letter gamma (Γ), the reflection coefficient describes how much of an electromagnetic wave is reflected by an impedance discontinuity such as a mismatched load. A slightly more obscure concept is that of the reflection coefficient. Radio operators are inherently familiar with the concept of standing-wave ratio, i.e., a measure of how well a load is matched to a transmission line. We’ll use an example to show how the Smith chart was developed. The Smith chart provides a concise way to view a number of different characteristics of RF systems, such as impedance and voltage standing-wave ratio (VSWR). In 1939, he had an article published in Electronics Magazine describing what later came to be known as the Smith chart. Fascinated by the repetitive nature of the impedance variation along a transmission line and its relation to the standing-wave amplitude ratio and wave position, Smith devised an impedance chart in which standing-wave ratios were represented by circles. Phillip Smith, 1ANB (SK), was an electrical engineer who graduated from Tufts College in 1928, and then went to work in the radio research department at Bell Telephone Laboratories. And that’s what ham radio’s all about, right? Otherwise, we may as well use a telephone or the Internet to communicate over large distances! And don’t worry we’ll keep the equations to a minimum (or in footnotes).
In addition, the knowledge gained in its use adds to our general knowledge of radio systems.
SMITH CHART VSWR SOFTWARE
Why use a seemingly-archaic tool such as the Smith chart, when calculators and software are readily available? For one, the chart remains useful as a visualization tool, representing a wealth of information in a very precise and intuitive manner. Here then, is a brief introduction to the Smith chart.
SMITH CHART VSWR FULL
But although questions regarding the chart appear in the Amateur Extra License exam, the current Question Pool has a mere 11 questions on the topic, leaving a full appreciation of the tool to the more inquisitive student. The advent of low-cost antenna analyzers and vector network analyzers (VNAs) has resulted in a renewed interest in an 80-year old tool called the Smith chart, a graphical device used to view the characteristics of RF transmission lines, antennas, and matching circuits, and to aid in the design of those systems.


 0 kommentar(er)
0 kommentar(er)
